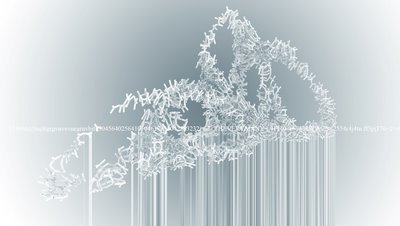
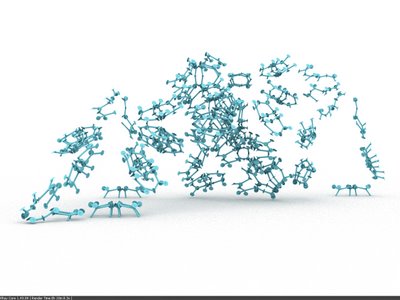
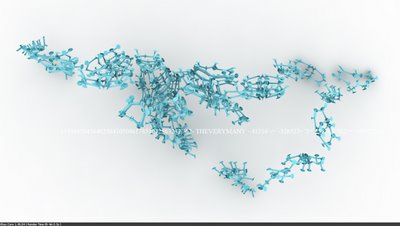
080406_APERIODIC VERTEBRAE v2.0.2

Finally back from several events and wanderings all over the place (Paris, New York, Frankfurt, Strasbourg, Barcelona,...) - here is a first update on the prototype THEVERYMANY produced for Node08 / Frankfurt.

Its assembly has this time been a success (and hughe improvement since v1.0) as it took less than 24 hours & 2 people & 2 laptops to (re-)assemble the 360 panels and 320 nodes...

Once more demonstrating us "one better spend its time within development embedding assembly logic rather than waiting the material world to solve the fuzzines..."

(I will update the "information modeling" improvements on the previous post more focused on the digital back bone approach of the piece...)




"Aperiodic Vertebrae v2.0"
THEVERYMANY (project team: Marc Fornes / Skylar Tibbits)
NODE08 (www.node08.vvvv.org)
April 5th - 12th, Frankfurt / Germany

Many thanks to Eno Henze (http://www.enohenze.de/) & the entire VVVV team (http://vvvv.org) for their invitation & sponsorship

Also many more thanks to our sponsors for the piece:

- Quadrant EPP USA, Inc. (www.quadrantepp.com) > provided us sheets of polyethylene (3/16″ thick)

- Continental Signs (www.continentalsigns.net) and Jared Laucks > CNC cut of the panels

- Dick Dunlop > laser cut of the 320 unique connections (3/16″ acryclic)

080131_Exhibition: Aperiodic_Vertebrae (day4)

"Generator.x 2.0: beyond the screen..." an exhibition curated by Marius Watz at the DAM(Berlin) with works by Jared Tarbell (US), Commonwealth (US), Theverymany (FR/US), Leander Herzog (CH), Marius Watz (NO) and participants of the Generator.x 2.0 Workshop...

THEVERYMANY (Marc Fornes, Skylar Tibbits) / Aperiodic_Vertebrae
LOG_assembly_day_04: things are going smoother - one day to go before the opening...

071117_Aperiodic_Series002

The flower is said to be the most conspicuous part of the plant. Their appeal has encouraged Man to know and possess them, developing technique such as gardening. The beauty of their petals - regarded as a highly modified leafs - has mainly been developed to attract pollinators (insects, birds or bats) which play an important role in the reproductive process of pollinating.

As an architect the easy shortcut of assimilating petals to cladding is a very tempting analogy: even though both have very different constraints and mode of operation, cladding -like petals- other than defining and protecting its host is often mainly regarded as an ornamental design exercise with one function only: made to attract… though within one rule only: within budget!



Here that shortcut has been taken to its paradigm as starting hypothesis: assuming the time of a geometrical wandering only - like some sort of temporary but controlled amnesia- that a cladding strategy could be elaborate on a flower attraction effect (affect??) though not by the complex geometry of its petal but rather by the intricacy of its assembly…

If “within a certain cost” intricacy can only be achieved within repetition - here:
- take 4 flowers (flower as assembly but also assemblage) describe within a pyramid
- each flower is made of 4 petals
- each petal is simplified based on a closed nurbs curve written within a triangle
- but also each petals is common at two flowers
- add 4 more flowers as the exact mirror of the first ones
You can therefore describe 8 different flowers of 4 petals with height 8 unique petals only…




If the entire story isn’t based on 4 random pyramids but based on four Danzer tiles you could depending on the scale potentially describe any shapes within such packing based on 4 flowers (connections) and 8 unique petals (tiles)…

071107_Aperiodic_Series001

Here is finally the second post on a series of tests done on 3d aperiodic pattern - here based on a Danzer tiling assembly - the other different types of outputs will be posted as a following series of post...

APERIODIC TILING (http://en.wikipedia.org/wiki/Aperiodic_tiling):
A given set of tiles, in the Euclidean plane or some other geometric setting, admits a tiling if non-overlapping copies of the tiles in the set can be fitted together to cover the entire space. A given set of tiles might admit periodic tilings, tilings that remain invariant after being shifted by a translation. (For example, a lattice of square tiles is periodic.) It is not difficult to design a set of tiles that admits non-periodic tilings as well (For example, randomly arranged tilings using a 2x2 square and 2x1 rectangle will typically be non-periodic.) An aperiodic set of tiles however, admits only non-periodic tilings, an altogether more subtle phenomenon.

DANZER TILING (http://www.cs.williams.edu/~98bcc/tiling/index.html):
There are 22 vertex configurations which occur in an infinite (global) Danzer tiling produced by inflating an initial finite patch an infinite number of times. Danzer says in his paper that there are 27 vertex configurations total, but says nothing about the characteristics of the five configurations which do not appear in a global tiling. We have identified a total of 174 vertex configurations by exhaustive search. At present we are unsure whether Danzer's remark is an error or whether some 5 of these are special in some way.



Acknowledgment: I can't pretend taking much credits in the field of aperiodic pattern in architecture as yet somehow in the direct line of people such as Daniel Bozia, Aranda/Lasch, K. Steinfeld and many others who posted on the web explicit and illustrated information on the subject which helped me to figured it out...