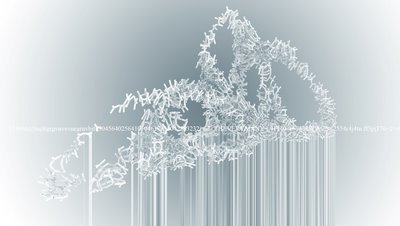
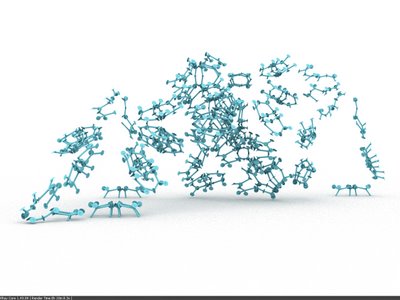
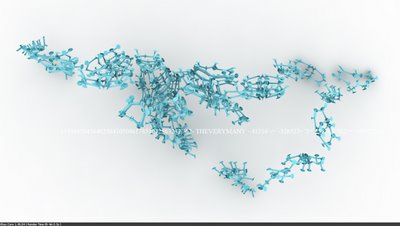
071117_Aperiodic_Series003_Gazebo

PAVILION - Free-standing structure (ie wikipedia)
Pavilion may refer to a free-standing structure sited a short distance from a main residence, whose architecture makes it an object of pleasure. Large or small, there is usually a connection with relaxation and pleasure in its intended use. A pavilion built to take advantage of a view is referred to as a gazebo.



POWERS OF TEN (ie wikipedia)
Powers of Ten is a 1977 short documentary film written and directed by Charles Eames and his wife, Ray. The film depicts the relative scale of the Universe in factors of ten (see also logarithmic scale and order of magnitude). The film is a modern adaptation of the 1957 book Cosmic View by Kees Boeke---and more recently is the basis of a new book version. Both adaptations, film and book, follow the the form of the Boeke original, adding color and photography to the black and white drawings employed by Boeke in his seminal work (Boeke's original concept and visual treatment is all too often uncredited or insufficiently credited in contemporary accounts).

The film begins with an aerial image of a man reclining on a blanket; the view is that of one metre across. The viewpoint, accompanied by expository voiceover by Philip Morrison, then slowly zooms out to a view ten metres across ( or 101 m in standard form), revealing that the man is picnicking in a park with a female companion. The zoom-out continues, to a view of 100 metres (10² m), then 1 kilometre (10³ m), and so on, increasing the perspective—the picnic is revealed to be taking place near Soldier Field on Chicago's lakefront—and continuing to zoom out to a field of view of 1024 metres, or the size of the observable universe. The camera then zooms back in to the picnic, and then to views of negative powers of ten—10-1 m (10 centimetres), and so forth, until we are viewing a carbon nucleus inside the man's hand at a range of 10-18 metre.






AA PAVILION "the powers of ten"
The different renders of that post are extracted from theverymany proposal for the AA Ten pavilion competition. The proposal was looking at "self-similarity" as the driving force behind the structure of its pavilion somehow allowing similarities within the possible "fractal" variation of the scale of its components -embeded within the logic of its aperiodic packing- and the emergent filiation between the 10 generations of AADRL graduates: all are different and all somehow within a certain depth are very similar...


Theverymany is now developping further its proposal -currently pushing with its aperiodic series- and is looking for sponsors and eventual venues to construct it - anyone interested?...

071117_Aperiodic_Series002

The flower is said to be the most conspicuous part of the plant. Their appeal has encouraged Man to know and possess them, developing technique such as gardening. The beauty of their petals - regarded as a highly modified leafs - has mainly been developed to attract pollinators (insects, birds or bats) which play an important role in the reproductive process of pollinating.

As an architect the easy shortcut of assimilating petals to cladding is a very tempting analogy: even though both have very different constraints and mode of operation, cladding -like petals- other than defining and protecting its host is often mainly regarded as an ornamental design exercise with one function only: made to attract… though within one rule only: within budget!



Here that shortcut has been taken to its paradigm as starting hypothesis: assuming the time of a geometrical wandering only - like some sort of temporary but controlled amnesia- that a cladding strategy could be elaborate on a flower attraction effect (affect??) though not by the complex geometry of its petal but rather by the intricacy of its assembly…

If “within a certain cost” intricacy can only be achieved within repetition - here:
- take 4 flowers (flower as assembly but also assemblage) describe within a pyramid
- each flower is made of 4 petals
- each petal is simplified based on a closed nurbs curve written within a triangle
- but also each petals is common at two flowers
- add 4 more flowers as the exact mirror of the first ones
You can therefore describe 8 different flowers of 4 petals with height 8 unique petals only…




If the entire story isn’t based on 4 random pyramids but based on four Danzer tiles you could depending on the scale potentially describe any shapes within such packing based on 4 flowers (connections) and 8 unique petals (tiles)…

071107_Aperiodic_Series001

Here is finally the second post on a series of tests done on 3d aperiodic pattern - here based on a Danzer tiling assembly - the other different types of outputs will be posted as a following series of post...

APERIODIC TILING (http://en.wikipedia.org/wiki/Aperiodic_tiling):
A given set of tiles, in the Euclidean plane or some other geometric setting, admits a tiling if non-overlapping copies of the tiles in the set can be fitted together to cover the entire space. A given set of tiles might admit periodic tilings, tilings that remain invariant after being shifted by a translation. (For example, a lattice of square tiles is periodic.) It is not difficult to design a set of tiles that admits non-periodic tilings as well (For example, randomly arranged tilings using a 2x2 square and 2x1 rectangle will typically be non-periodic.) An aperiodic set of tiles however, admits only non-periodic tilings, an altogether more subtle phenomenon.

DANZER TILING (http://www.cs.williams.edu/~98bcc/tiling/index.html):
There are 22 vertex configurations which occur in an infinite (global) Danzer tiling produced by inflating an initial finite patch an infinite number of times. Danzer says in his paper that there are 27 vertex configurations total, but says nothing about the characteristics of the five configurations which do not appear in a global tiling. We have identified a total of 174 vertex configurations by exhaustive search. At present we are unsure whether Danzer's remark is an error or whether some 5 of these are special in some way.



Acknowledgment: I can't pretend taking much credits in the field of aperiodic pattern in architecture as yet somehow in the direct line of people such as Daniel Bozia, Aranda/Lasch, K. Steinfeld and many others who posted on the web explicit and illustrated information on the subject which helped me to figured it out...